What Is The Volume Of A Sphere With A Radius Of 6 Mm

These settings emphasize how the derivative enables us to quantify how the quantity \(y\) is changing as \(x\) changes at a given \(x\)-value. Through knowing how the quantities are related, we will be interested in determining how their respective rates of change with respect to time are related. For example, suppose that air is being pumped into a spherical balloon in such a way that its volume increases at a constant rate of 20 cubic inches per second.

More specifically, can we find how fast the radius of the balloon is increasing at the moment the balloon's diameter is 12 inches? The following preview activity leads you through the steps to answer this question. If you ever wondered what's the volume of the Earth, soccer ball or a helium balloon, our sphere volume calculator is here for you.

It can help to calculate the volume of the sphere, given the radius or the circumference. Also, thanks to this calculator you can determine the spherical cap volume or hemisphere volume. Find the volume of water left in the cylinder, if the radius of the cylinder is 60 cn and its height is 180 cm.

Conical and pyramidal shapes are often used, generally in a truncated form, to store grain and other commodities. Similarly a silo in the form of a cylinder, sometimes with a cone on the bottom, is often used as a place of storage. It is important to be able to calculate the volume and surface area of these solids. Calculating volume and surface area of sphere play an important role in mathematics and real life as well. Formulas for volume & surface area of sphere can be used to explore many other formulas and mathematical equations.

A water tank has the shape of an inverted circular cone with a base of radius 6 feet and a depth of 8 feet. Suppose that water is being pumped into the tank at a constant instantaneous rate of 4 cubic feet per minute. Now the question becomes calculating the volume of the bicylinder . It is also very difficult, so add a cube packing the bicylinder .

Now when the plane intersects the cube, it forms another larger square. The extra area in the large square , is the same as 4 small squares . Moving through the whole bicylinder generates a total of 8 pyramids. Recognizing familiar geometric configurations is one way that we relate the changing quantities in a given problem. For instance, while the problem in Activity \(\PageIndex\) is centered on a conical tank, one of the most important observations is that there are two key right triangles present.

In another setting, a right triangle might be indicative of an opportunity to take advantage of the Pythagorean Theorem to relate the legs of the triangle. Q.9.A wooden article was made by scooping out a hemisphere from each end of a solid cylinder, as show in Fig. If the height of the cylinder is 10 cm, and its base is of radius 3.5 cm, find the total surface area of the article. This statement is not at all obvious or elementary. "A sphere's volume is two cones of equal height and radius to that of the sphere's".

The assertion about the cone and the cylinder is a little easier to prove, but it too is not obvious. So you have not really provided an answer to this to year old question. I think the accepted answer is closest to what you have in mind.

If you want to help here I think you should pay attention to new questions that don't yet have answers. A hollow sphere of internal and external radii 2 cm and 4 cm respectively is melted into a cone of base radius 4 cm. Find the height and slant height of the cone. The surface area of a solid metallic sphere is 616 cm2.

It is melted and recast into a cone of height 28 cm. Find the diameter of the base of the cone so formed. A solid metallic sphere of radius 10.5 cm is melted and recast into a number of smaller cones, each of radius 3.5 cm and height 3 cm. Find the number of cones so formed.

In this module, we will examine how to find the surface area of a cylinder and develop the formulae for the volume and surface area of a pyramid, a cone and a sphere. These solids differ from prisms in that they do not have uniform cross sections. The radius of the internal and external surfaces of a hollow spherical shell are 3 cm and 5 cm respectively.

If it is melted and recast into a solid cylinder of height 22/3 cm. Find the diameter of the cylinder. This online calculator will calculate the 3 unknown values of a sphere given any 1 known variable including radius r, surface area A, volume V and circumference C. It will also give the answers for volume, surface area and circumference in terms of PI π. A sphere is a set of points in three dimensional space that are located at an equal distance r from a given point . It is a straightforward question, but the visual does not make it clear that the inner sphere is empty especially when the text of the question doesn't make it apparent.

I was stuck on it for a while on GMATPrepExam, then assumed that the question is asking for the difference in volume adjusted weight. It is surprising because in PS questions, the stem is generally very clear and without any ambiguity. For example, even in the same question - formula of a sphere is provided. Most of the times, PS questions don't mind giving redundant information through text that is already there in the pic. The variation in density and crustal thickness causes gravity to vary across the surface and in time, so that the mean sea level differs from the ellipsoid. This difference is the geoid height, positive above or outside the ellipsoid, negative below or inside.

The geoid height variation is under 110 m on Earth. The geoid height can change abruptly due to earthquakes (such as the Sumatra-Andaman earthquake) or reduction in ice masses . Q.1.A solid is in the shape of a cone standing on a hemisphere with both their radii being equal to 1 cm and the height of the cone is equal to its radius. Find the volume of the solid in terms of π. Q.5.A hemispherical depression is cut out from one face of a cubical wooden block such that the diameter l of the hemisphere is equal to the edge of the cube.

Determine the surface area of the remaining solid. Q.2.A vessel is in the form of a hollow hemisphere mounted by a hollow cylinder. The diameter of the hemisphere is 14 cm and the total height of the vessel is 13 cm. Find the inner surface area of the vessel. The diameters of the internal and external surfaces of a hollow spherical shell are 6 cm and 10 cm respectively. If it is melted and recast into a solid cylinder of diameter 14 cm, find the height of the cylinder.

A solid metallic sphere of radius 5.6 cm is melted and solid cones each of radius 2.8 cm and height 3.2 cm are made. Find the number of such cones formed. Volume is the amount of total space on the interior of the solid.

Knowing the definition of volume, we can now focus on the formulas for volume of common geometric solids. Using these formulas manually won't be difficult, but for fast, accurate results every time, use the volume calculator. A glass dome for a lighting fixture is in the shape of a hemisphere. The circumference of the great circle of the hemisphere is 12π inches. Which statements about the hemisphere are true?

The total surface area is 108π square inches. The total surface area is 144π square inches. The total surface area is 432π square inches. The total surface area is 36π square inches. The baseball is not regulation size. If the baseball has a surface area of 9π, then I can set that equal to the formula 4πr2 and solve for r.

The radius is 3/2 inches, so I double that to find the diameter. The diameter of the ball is 3 inches, which is greater than the allowed range of diameters. The volume of a sphere formula can be found in terms of the diameter. The diameter of a sphere is the longest line that is inside the sphere and that passes through the center of the sphere. A sphere is a three-dimensional shape that is perfectly symmetrical and round in shape.

Some examples of spheres are a ball, a globe, etc. The volume of a sphere is the amount of space that is inside it the capacity of the sphere that it can hold. In this article, we will derive the diameter of a sphere formula using the volume.

In this lesson, you'll learn how to find the volume of a sphere with a radius of 4 inches. A sphere is a 3-dimensional round object. The volume is how space is inside the sphere. When you want to find out how much space is inside such a sphere, you'll follow these steps to calculate the volume of the sphere. For any other value for the length of the radius of a sphere, just supply a positive real number and click on the GENERATE WORK button. They can use these methods in order to determine the surface area and volume of parts of a sphere.

A set of points in a space equally distanced from a given point $O$ is a sphere. The point $O$ is called the center of the sphere. The distance from the center of a sphere to any point on the sphere is called the radius of this sphere. A radius of a sphere must be a positive real number. The segment connecting two points on the sphere and passing through the center is called a diameter of the sphere.

All radii of the sphere are congruent to each other. A sphere can be obtained by rotating a semicircle about the diameter. Two spheres of the same radius are congruent.

Draw several spheres with different radii, and observe that as volume changes, the radius, diameter, and surface area of the balloon also change. Jeremy has a large cylindrical fish tank that he bathes in because he doesn't like showers or bathtubs. The following video shows how to solve problems involving the formulas for the surface area and volume of spheres. Q.2.Metallic spheres of radii 6 cm, 8 cm and 10 cm, respectively, are melted to form a single solid sphere.

Find the radius of the resulting sphere. Q.1.A metallie sphere of radius 4.2 cm is melted and recast into the shape of a cylinder of radius 6 cm. Q.8.From a solid cylinder whose height is 2.4 cm and diameter 1.4 cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid to the nearest cm2. The volume calculation is easy for regular figures, such as cubes, rectangular boxes and pyramids, because all you need to do is measure the dimensions and use a formula.

In these calculations, we assumed a spherical shape for the nanoparticles. As a geometric object the sphere has the smallest of all surface areas. Real nanoparticles may be semi-spheroidal, but they also have every imaginable shape from potato-shaped to pancake-shaped. In Part 1 we noted that nanomaterials may take the form of tubes, wires and sheets.

All these shapes have surface areas that are even larger per particle than the ones we calculated for spheres. That is to say a nanotube consisting of 1000 atoms has a larger surface area than a sphere of 1000 atoms. A spherical ball of radius 3 cm is melted and recast into three spherical balls. The radii of two of the balls are 1.5 cm and 2 cm.

Find the diameter of the third ball. An iron spherical ball has been melted and recast into smaller balls of equal size. If the radius of each of the smaller balls is 1/4 of the radius of the original ball, how many such balls are made? Compare the surface area, of all the smaller balls combined together with that of the original ball.

The diameter of a metallic sphere is equal to 9 cm. It is melted and drawn into a long wire of diameter 2 mm having uniform cross-section. Take a hemisphere of radius and look at the area of a typical cross-section at height above the base. A sphere, a cylinder, and a cone have the same diameter. The height of the cylinder and also the cone are equal to the diameter of the sphere. A cone and a hemisphere have equal bases and equal volumes.

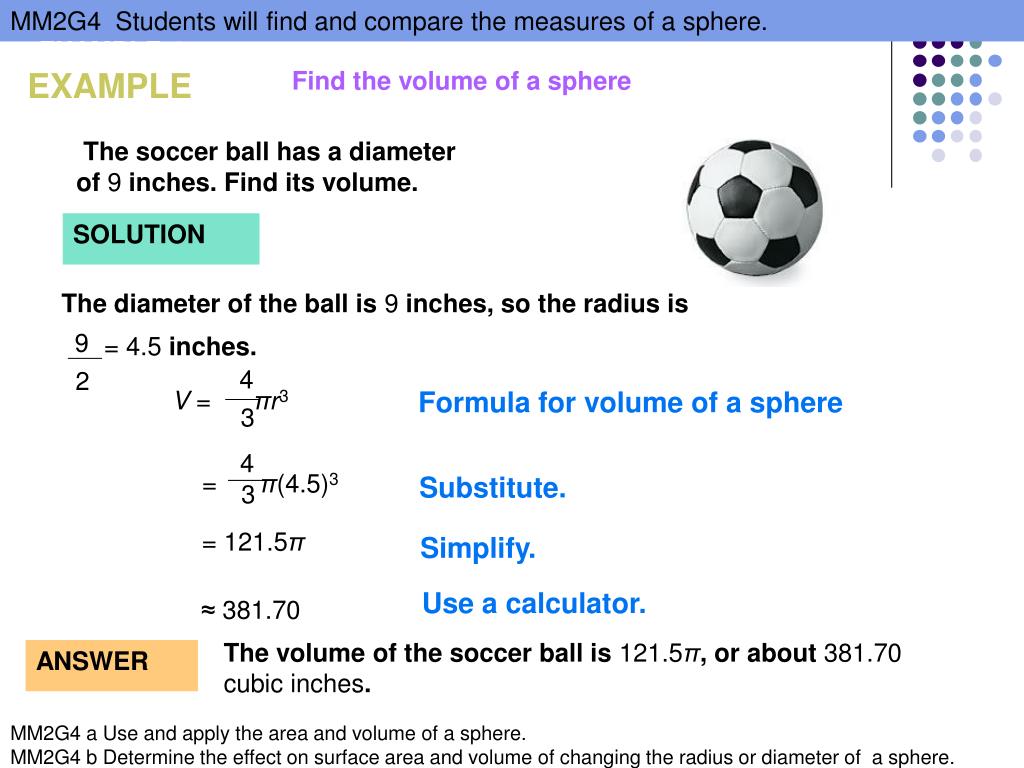
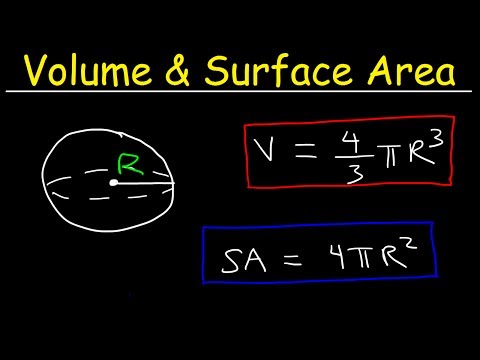
There is another special formula for finding the volume of a sphere. The volume is how much space takes up the inside of a sphere. The answer to a volume question is always in cubic units. The formula for the volume of a sphere is 4/3 times pi times the radius cubed.